Vvpf

VVPF 3.x

RHEOLOGY OF PARTICLE SUSPENSIONS

Theme:

The material model used in Viscometric-ViscoPlastic-Flow 3.x is named the PFI-theory (Mark II). The theory consists of system of coupled partial differential equations (PDEs). The model has quite many material parameters which are not directly observable and hence are generally unknown. VVPF 3.x has a search module that can automatically retrieve the values of these model parameters. This is done by combining computational fluid dynamics (CFD) with a certain statistical tool, called the direct search method [2]. The overall algorithm solves an optimization problem, in which the difference between the observed data and the output of the PDEs is minimized.

Automatic parameter identification of linear algebraic equation is straightforward, where equation like y = ax + b is fitted to the observed data. That is, through the method of least squares for example, the parameters a and b are easily extracted. However, automatic parameter identification of system of PDEs is much more challenging, basically because there is generally no algebraic solution to the equations. That is, there exist only numerical solutions to the PDEs. Also, the problem of parametric identification is generally difficult due to both the number of parameters that a model can rely on and due to the nonlinear behavior of the governing PDEs. In vvpf 2.0 and 1.0 (see [3,4]), such parameter identification was done manually, where all values were extracted by the means of manual trial and error. That is, all values were manually changed by a computer operator, until the computed data more or less overlapped the measured data. Since automatic parameter identification can play a key role in examining the validity of a complex material model, the generality of the current work is beyond the specific model presented in vvpf 3.x.

The manual / documentation for this software (in its current working state) can be found here. Please note that the document is in its infancy, so there are lot of rough spots to fix.

BIBLIOGRAPHY

1. Unpublished!
2. J.E. Wallevik, Method of Retrieving the Herschel-Bulkley Parameters When Using the (Wide-Gap) Coaxial Cylinders Viscometer, The Third North American Conference on the Design and Use of Self-Consolidating Concrete, November 10-12, 2008, Chicago, IL.
3. J.E. Wallevik, Rheological properties of cement paste: thixotropic behavior and structural breakdown, Cement and Concrete Research, 39 (2009) 14 - 29.
4. J.E. Wallevik, Thixotropic investigation on cement paste: experimental and numerical approach, J. Non-Newtonian Fluid Mech. 132 (2005) 86 - 99.

VVPF 2.0

RHEOLOGY OF PARTICLE SUSPENSIONS

Theme:

The material model used in Viscometric-ViscoPlastic-Flow 2.0 is named the PFI-theory (Mark I) and consists of system of coupled partial differential equations (PDEs). In the earlier work (vvpf 1.0), the material model was only thixotropic in origin, based on several ideas proposes by Hattori and Izumi. With a purely thixotropic characteristic, the model was only partly successful. In the model present in vvpf 2.0, however, a certain time-dependent and non-thixotropic process, called structural breakdown is allowed to occur simultaneously with the thixotropic behavior. The combination of the two processes produces a much better result. The concept of structural breakdown is from previous work done by Tattersall and Banfill.

The manual / documentation for this software (in its current working state) can be found here. Please note that the document is in its infancy, so there are lot of rough spots to fix.

BIBLIOGRAPHY

1. J.E. Wallevik, Rheological properties of cement paste: thixotropic behavior and structural breakdown, Cement and Concrete Research, 39 (2009) 14 - 29.

VVPF 1.0

RHEOLOGY OF PARTICLE SUSPENSIONS - Fresh Concrete, Mortar and Cement Paste with Various Types of Lignosulfonates

Download:

http://www.diva-portal.org/ntnu/theses/abstract.xsql?dbid=319 (in PDF format)

Theme:

Viscometric-ViscoPlastic-Flow 1.0 is actually two different programs. The first one (vvpf10_ct34) solves the flow of viscoplastic material inside the ConTec BML Viscometer 3 (see Figure 8.15, page 197) and ConTec Viscometer 4 (see Figure 9.5, page 217), both for steady state and transient cases. The second software (vvpf10_c3p2) calculates the flow of a viscoplastic material inside a modified cone viscometer, called C3P2 (see Figure 10.30, page 264). For the latter software, only steady state cases are calculated.

1. Chapter 7 - Computational Rheology: Gives an introduction to the finite difference method. It is used in VVPF for solving the governing equation. Calculation of shear rate is treated here. Consistency and iteration scheme are also treated in this chapter.
2. Chapter 8 - Simulation of the ConTec Viscometers: The setup of the auxiliary (boundary and initial) conditions used for the ConTec viscometers. Other issues like convergence and stability are also discussed in Chapter 8.
3. Chapter 9 - Thixotropic Explorations: Example of how VVPF 1.0 can be used.
4. Section 10.3.7 - The C3P2-Geometry: The treatment and implementation of a new geometry for the ConTec viscometers.
5. Appendix A - Source Code: Here the source code is shown. This code can also be downloaded in the download section.

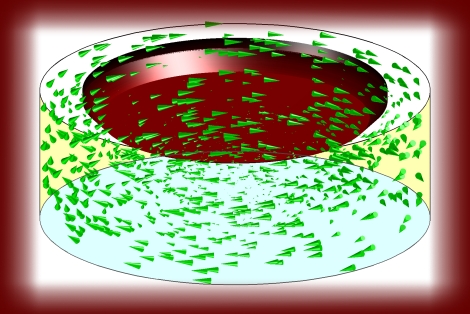
The above figure is from page 264 (Figure 10.30). It shows the velocity profile inside a modified cone viscometer. For this figure, a Bingham fluid is applied with yield value and plastic viscosity of 200 Pa and 20 Pa.s, respectively. The top red region is the measuring unit and is stationary, while the rest of the boundary consist of a rotating bucket (here, rotating at 3 rad/s).

BIBLIOGRAPHY

1. Wallevik, J. E., Minimizing end-effects in the coaxial cylinders viscometer: Viscoplastic flow inside the ConTec BML Viscometer 3. J. Non-Newtonian Fluid Mech. 155 (2008) 116-123.
2. Wallevik, J. E., Thixotropic investigation on cement paste: Experimental and numerical approach. J. Non-Newtonian Fluid Mech. 132 (2005) 86-99.
3. Wallevik, J. E. (2004); Microstructure-Rheology: Thixotropy and Workability Loss; Nordic Concrete Research, Publication No. 31, The Nordic Concrete Federation 1/2004, Norsk Betongforening, Oslo, Norway.
4. Wallevik, J. E. (2003); Rheology of Particle Suspensions - Fresh Concrete, Mortar and Cement Paste with Various Types of Lignosulfonates (Ph.D.-thesis); Department of Structural Engineering, The Norwegian University of Science and Technology. ISBN 82-471-5566-4; ISSN 0809-103X.